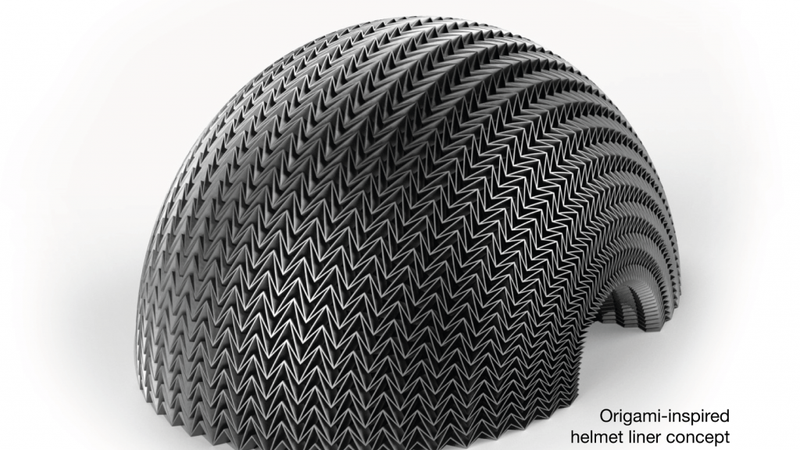
Control the collapse: Architected materials for impact absorption
When it comes to absorbing an impact, something’s got to give. Generally, that is one of two things collapsing: a protective structure like a helmet (which is hopefully present), or the sensitive object being protected (e.g. a person’s body or head).
This article was first published on
ntopology.comIntroduction
When it comes to absorbing an impact, something’s got to give. Generally, that is one of two things collapsing: a protective structure like a helmet (which is hopefully present), or the sensitive object being protected (e.g. a person’s body or head). Fortunately, engineers have some influence over the former, and can control the structure’s deformation through design to absorb the impact energy. This level of design control is rapidly expanding with technologies such as additive manufacturing. Lattices and cellular structures, or architected materials, in particular offer a vast design space to tune and optimize this deformation capability and resulting energy absorption capacity. This post will attempt to cover a few of the basic design principles with regards to additively manufactured architected materials for impact protection.
Going with the grain
Just as woods and fiber composites have a distinct grain or bias to them, and resultant directional properties (i.e. anisotropy), so do architected materials. In woods, the difference in compressive strength when oriented along or against the grain can differ by an order of magnitude [1]. Fiber composites allow the engineer to carefully control properties in-plane by strategically orienting the fabric layers, but requires them to take care to design the overall structures to avoid out-of-plane loading where they tend to delaminate.
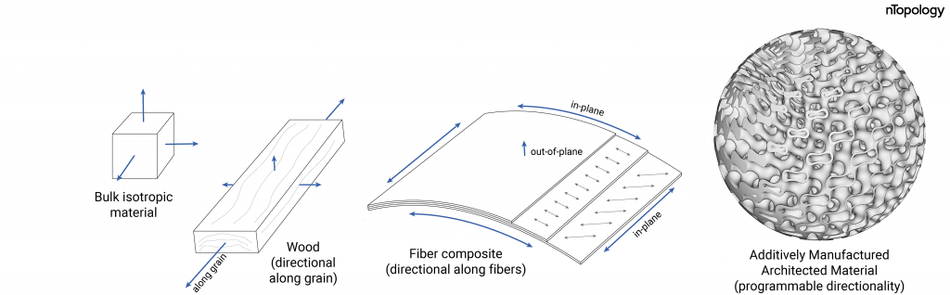
Additively manufactured lattices, however, are the ultimate in customization potential for directional properties — the only limitations being the manufacturing process, design tools, and engineer’s imagination. A single unit cell can not only be tuned to morph its material properties as desired (see Figure 2), but spatially varied through the part to locally tailor to the engineer’s specifications. This quickly becomes an overwhelmingly multidimensional design space. Where do we even begin?
Slow motion
The ability to freely tune material properties in 3D allows the engineer to architect their own material properties to fit their application. With enough unit cells in the lattice, one can essentially treat the structure as a special new effective material. A visual representation of this on a unit-cell level can be seen below, where the grey is the physical unit cell to be manufactured, and the colored shape is an indication of its directional material properties.

This design process assumes quasi-static loading. Will these properties change under impact conditions? Absolutely. Many base materials are strain-rate sensitive, and exhibit different properties under different loading rates. In this post, we are just focusing on the architecture, not the chemistry and micro-mechanics of the base material from which it is made. In a real design process, an engineer has probably already down-selected a few materials which they can print, which focuses the design decisions on the architecture anyways. In a similar line of thinking, they have probably selected a temperature range too (in addition to strain-rates, materials behave very differently at different temperatures). Focusing primarily on the architecture allows an apples-to-apples comparison, and can get us surprisingly far.
Falling apples
Speaking of apples, even the lightest of lattices cannot escape from Newton’s Laws of Motion. Every strut or element in the architected material has inertia associated with it, and will remain at rest unless some force acts upon it. The intricate arrangements of material (i.e. mass) in these structures can make for a spring-like maze for forces and shocks to traverse, and contributes to what is known as inertial sensitivity of the structure.
Calladine & English first described these effects as “strain-rate” (i.e. material) and “inertia effects” (i.e. topology/architecture) in 1984 [2]. Interestingly, they discovered these trends in preparation of a drop-testing program for an undergraduate laboratory course at Cambridge, and it became a much more detailed topic of study at the university afterwards [3].

Notice the force-displacement and energy-displacement (i.e. the integral) response for the two types of structures above in Figure 3. Type II has a strong start and absorbs energy early, but then softens rapidly as the structure buckles and collapses. Type I is weaker at first but maintains this steady plateau throughout the collapse. Perhaps one can see in Figure 4 how this can extend to architected materials, and how it might even vary within the same structure.
As any great experimentalists would, they dropped heavy weights on the specimens. Upon application of the same energy, the curved Type I structure crushed a lot further than the kinked Type II, with the straighter walls of the latter putting up more inertial resistance to the impact load [2]. The reason can be simplified into something rather intuitive: generally, the more material aligned with the direction of loading, the more mass there is to redirect (or inertia) in order to crush it.
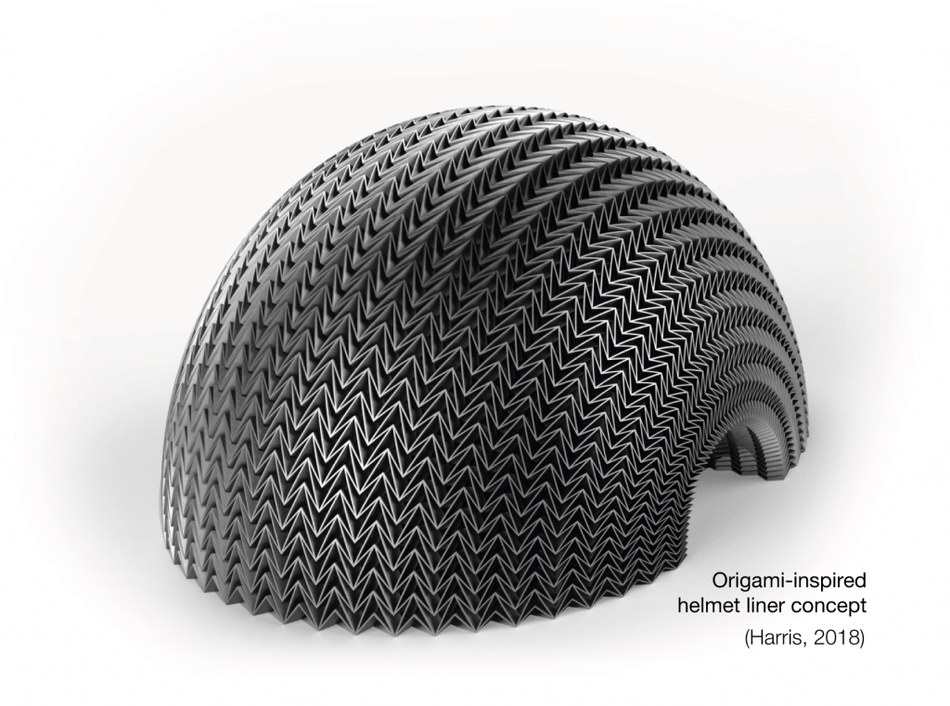
I conducted a few experiments and simulations inspired by these (albeit many years later) on some additively manufactured origami specimens, and found that with the right unit cell the degree of Type I or Type II behavior can be freely varied based on aligning this material with the loading direction [4].

Inertial sensitivity means that the structure will perform differently under impact conditions. Indeed, the strength, energy absorption, and even deformation mechanisms can vary significantly compared to quasi-static compression. However, as alluded to earlier, the quasi-static response can get us surprisingly far, even in making predictions in the dynamic world. The force-deflection response can often be used to predict whether a structure is Type I or Type II, and thus whether strain-rate or inertial effects will be dominant. Oftentimes with a stiff and strong unit cell architecture, one finds a more inertially sensitive structure. With a softer more foam-like configuration, inertia is less of a factor, and strain-rate sensitivity of the material may be more of a consideration. Of course, this behavior is also anisotropic as in Figure 2. Designing with architected materials inherently requires many iterations to optimize.
Conclusion
There are plenty more factors at play in designing protective structures: from physics (material strain-rate sensitivity, fatigue over repeated impact cycles, off-angle threat protection, blunt impact vs. penetration resistance, etc.) to human factors (helmet fit, environmental conditions, or if it is even worn). However, equipped with the right tools, an engineer can have substantial control over the anisotropy and inertial sensitivity in architected materials, and use these characteristics to their advantage in designing their parts. An understanding of these two factors will build the foundation for optimizing the rest.
References
[1] J.J. Harrigan, S.R. Reid, P.J. Tan, and T. Yella Reddy, “High rate crushing of wood along the grain,” International Journal of Mechanical Sciences, 2005. https://doi.org/10.1016/S0734-743X(97)00016-X
[2] C. Calladine and R. English, “Strain-rate and inertia effects in the collapse of two types of energy-absorbing structure,” International Journal of Mechanical Sciences, 1984. https://doi.org/10.1016/0020-7403(84)90021-3
[3] L. Tam and C. Calladine, “Inertia and strain-rate effects in a simple plate-structure under impact loading,” International Journal of Impact Engineering, 1991. https://doi.org/10.1016/0734-743X(91)90044-G
[4] J.A. Harris (2018). Additively Manufactured Metallic Cellular Materials for Blast and Impact Mitigation (Doctoral thesis). https://doi.org/10.17863/CAM.18766
This article was first published on the nTopology blog.