Jumping robot
An engineered jumper uses work multiplication with hybrid tension-compression spring linkage to achieve heights over 30 meters.
Technical Specifications
| Transmission | Spring-actuated |
| Selected scale: 0.3 m | |
| Motor | Small rotary motor (10 g) |
| large gear reduction (1,000:1) | |
| Tension: 130 N | |
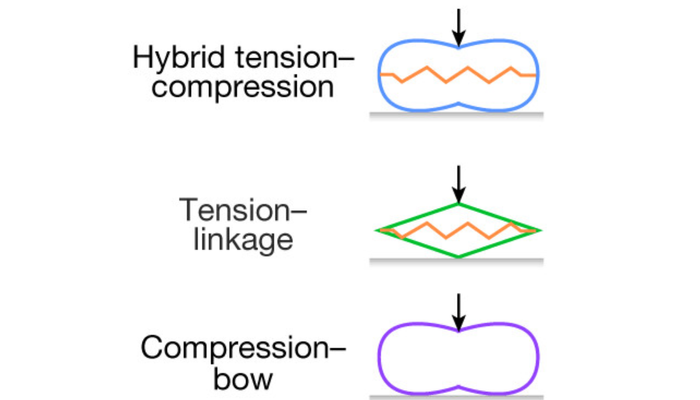
| Linkage | Hybrid tension–compression spring-linkage |
| Spring | Materials: carbon fibre and latex rubber |
| Rod width-to-length ratio: ~0.017 | |
| Rod thickness-to-length: ~0.0054 | |
| Ties width-to-length ratio: ~0.11 | |
| Sweet Spot Thickness-to-length ratio: 0.073 | |
| Rods | Material: carbon-fibre composite |
| Nominal fibre volume: 65% | |
| Tensile strength: 1.7 GPa | |
| Tensile modulus: 140 GPa | |
| Flexural strength: 1.8 GPa | |
| Flexural modulus: 130 GPa | |
| Ultimate tensile strain: 1.9% | |
| Density: 1.56g/cm3 | |
| Ties | Material: latex rubber |
| Tensile strength: 3 MPa | |
| Modulus (approximated as constant): 300 kPa | |
| Ultimate tensile strain: 660% | |
| Density: 0.74g/cm3 | |
| Compression bow | 1,922 J kg−1 |
| strain value: 1.6% | |
| thickness-to-length aspect ratio: 0.0051 | |
| width-to-length aspect ratio: 0.026 | |
| Spring–motor mass ratio (spring-linkage mass: 12.4 g) | 1.2 (vs 0.025–0.06 in biological jumpers) |
| Payload-free specific energy | 1,075 J kg−1 (24.2 J per 22.5 g) |
| Acceleration | 0 to over 28 m s−1 in 9 ms (>3,000 m s−2) |
| Time-of-flight (3 jumps) | 5.13, 5.18, 5.25 s |
| Achieved heights (3 jumps) | 32.2, 32.8, 33.6 m |
| Average height | 32.9 ± 0.7 m (±s.d.) |
| Inclined jump | Angle: 45° |
| Height: 17 m | |
| Length: 50 m |
Overview
Problem / Solution
Engineers have mimicked nature's art of biological jump while scientists try to explore the limits of this natural wonder. While efforts reach certain success, there are no general analyses comparing the key differences in the energetics between biological and engineered jumpers. Biological jumpers depend their jump height on their muscle that causes linear motion in a single stroke, whereas engineered devices work on the principle of rotary motor that multiplies work to increase the jump height. Despite this notable difference, no general modeling framework exists to quantify such differences.
Engineered motors are capable of overcoming the single-stroke work limit of biological motors. In linear motors, ratchets can combine multiple strokes, while in rotary motors, repeated turns combine multiple rotations to cause ‘work multiplication’. This event is only possible in engineered jumpers since ratchets and motors do not exist on biology’s cellular scale. The insights from such models allow researchers to extend the limits in specific-energy production, using electromagnetic motors in engineered devices that can jump over 30 meters.
Design
Model
A jump is a movement caused by forces exerted on the ground by a jumper, maintaining constant mass during the process. Two aspects analyzed in a jump are specific production limits, defined as the maximum energy created in a single jump per unit mass of the jumper, and specific energy utilization or the conversion efficiency of the specific energy to a jump height.
In work multiplication, increasing the gear reduction between the overall motion of the jumper and the motor stroke also increases the number of rotations. Work multiplication in direct-drive transmission occurs during the acceleration phase, and during the pre-stretch phase in spring-actuated transmission. It is only possible in engineered jumpers since the ratchet and rotary motor types have not been seen in biology yet.
There are three primary limiters in describing the upper limits of specific-energy production. The first limiter, which does not exist in engineered jumpers, is the single-stroke specific work. Work multiplication relieves this integral of specific force over stroke. The second limiter, coined as motor power limiter, is the product of power and acceleration time or the motor’s specific power time. The spring-specific energy dictates the third limiter, it is the amount of energy the string stores and releases per unit mass. The spring energy limiter exists on spring-actuated transmissions only.
Model results and insights
Findings demonstrate that the motor work limiter can never be exceeded by biological jumpers. But it can be achieved by artificial jumpers as work multiplication removes the motor work limiter for engineered jumpers. With an upper limit imposed by the spring energy limiter and the linkage mass, spring-actuated transmissions produce larger jumps than direct-drive transmissions at a small scale. At large scales, the motor power limiter and linkage mass impose an upper bound directing the superiority of direct-drive transmissions. Thus, as a result of these disparities in energetics, biological and artificial jumpers should have different designs for maximizing specific-energy production—and likewise in the jump height limit.
These design differences provide three key insights. First, for biological jumpers, the crossover scale is approximately 1 m (0.6 s acceleration time) while for engineered jumpers, the scale records two orders of magnitude greater at 100 m (3 s acceleration time).
Second, compared to biological jumpers, engineered jumpers must have a much higher ratio of spring to motor mass. Motor work becomes a limiting factor in biological systems. To overcome this, the spring energy capacity must be equal to but not more than the motor work. Given the larger specific energy needed comparing to the motor work, a much smaller motor spring mass will suffice the need. Likewise, in engineered jumpers, work multiplication causes large amounts of energy stored in the spring—requiring a much larger spring-motor mass ratio than in biological systems. Work multiplication also allows for better utilization of high specific energy in springs.
Third, for spring-actuated transmissions, maximizing of parameters between the biological and engineered jumpers depends on the primary limiter of their specific energy: specific motor work for biological, and spring plus linkage’s combined specific energy for engineered jumpers.
Jumper design
These insights lead to going beyond the limits of specific-energy production, using electromagnetic motors for engineered devices. A spring-actuated transmission with superiority at a scale of 0.3 m prompts the vision for a higher and more stable jump. The spring-motor ratio set to a higher value uses a small rotary motor (10 g) with a large gear reduction (1,000:1), enabling motor compression of a 130N tension spring wrapped around the spindle.
To overcome peak force constraint, a high specific-energy hybrid spring-linkage is designed through a custom nonlinear simulation framework. The hybrid tension-compression spring linkage made from carbon fibre and latex rubber supports the rubber in tension with a compression bow at 1,922 J kg−1. The tension linkage now stores energy and is no longer passive, whereas the compression bow now has a high-specific-energy rubber in tension. The spring linkage also exhibits a nearly constant force-displacement curve, helping store larger amounts of energy despite the force constraint. The spring-motor mass ratio scores 1.2 as compared to biological jumpers’ 0.025–0.06.
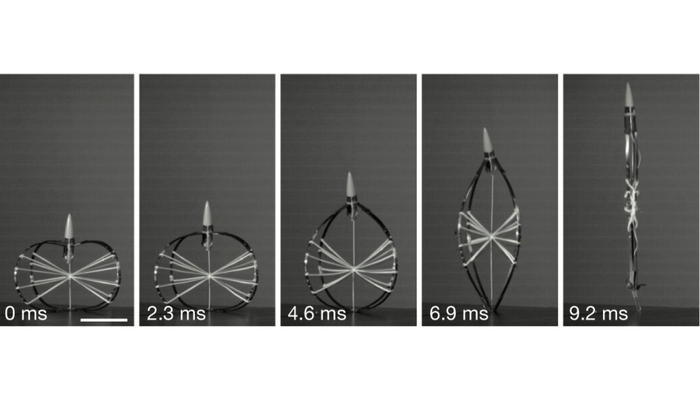
The hybrid spring linkage with its improved motor design fashions a jumper with minimal losses across six stages of energy utilization. For one, minimizing the mass of the ‘foot’ reduces the energy transfer loss while creating a shape-changing morphology that minimizes air drag. The payload-free specific energy caps at 1,075 J kg−1, where the 30-gram jumper accelerates from 0 to over 28 m s−1 within 9 ms. The jumper can reach a height of 32.9 ± 0.7 m (±s.d.).
References
This video shows a jumper taking off in slow motion (1/10, 1/100, and 1/500× real time). Take-off occurs in 9 ms after latch release.
This video shows a device jumping over 30 m high in an outdoor test. The person is 1.83 m tall.